Poincare' conjecture

I feel like I should say something meaningful since Grigory Perelman solved the Poincare Conjecture which states that any closed simply connected 3-dimensional manifold is homeomorphic to the standard 3-dimensional sphere.

Without going into homology, I'll try to explain in laymen's terms...Basically any 3-d bounded blob (ex. no feelers going off into infinity) living in 4-d can be collapsed into a sphere without ripping, tearing or ajoining. Yeah, that was a bad attempt. There's a reason why I don't teach anymore! The Clay Mathematics institute is much more eloquent than I.

You're probably going, oh come on, how complicated can a blobby thing be? Heh. I present to you the Alexander Horned Sphere:

This happens to be one of my favorite topological verities.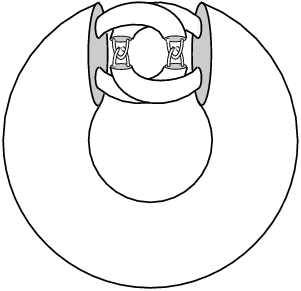
It's a fractal (a type of Cantor set to be exact), so as you zoom into those horny parts, you see more detail. This blob is homeomorphic to a sphere.

I think its interesting the conjecture has been proven for higher dimensions prior to Perelman's contribution for n=3. Humans are 3 dimensional creatures coexisting with time, in a 4 dimensional space. Perhaps it was because we live too close akin to the objects in question which is why n=3 alluded mathematicians for so long.

**
To the person who said bunnies are the same as spheres....That's not true! The digestive track runs from mouth to anus, so they are tori. Okay, I'm done ranting now.